Not only are these outstanding football teams, but three of the four teams featured three of the game's best quarterbacks in Manning, Favre and Brees. I thought it would be illustrative to take a look at each of the three quarterbacks individually, and then compare the three side by side to get a sense who maybe better, and perhaps even, who may be best. In evaluating their relative merits, I will only consider their ability to throw the football, not win games, or how well they run, or whether they are clutch performers, or how many Super Bowl victories they have.
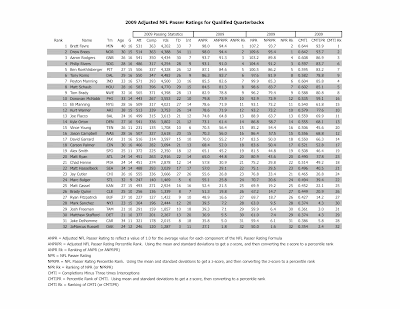
Here are the career NFL Passer Ratings for these quarterbacks:
Manning is clearly the best. Well, at least that's what looking at the career NFL Passer Rating will tell you. These figures differ from what you would find at the Pro Football Hall of Fame (as of January 23rd, 2010). The reason being that the figures listed on the Pro Football Hall of Fame site were as of the beginning of the 2009 season, and mine are as of the end of the 2009 season just completed.
For reasons that I have chronicled in the past (see here, here, and here), the NFL Passer Rating formula is in and of itself is of little use in determining who among the three might be best. The three quarterbacks very well might end up being ranked this way when it is all said and done, but that doesn't justify the rating system.
In order to complete our analysis, we have to make several adjustments to the NFL's passer rating system.
The first, is that we need to take each player's passer rating, and relate it to some baseline, so that we may account for players playing in different eras (yeah, I know, it's a little bit odd to be talking about players in different eras when comparing three current quarterbacks, but Brett Favre has been throwing since 1992, and Drew Brees only since 2002. And since the average NFL passer rating has been increasing over time (see here), we need to make an adjustment for that. The method that I have chosen is to relate a given quarterback's performance relative to the mean for that year, and use the standard deviation in that year as the measuring stick. In other words, the difference between the quarterback's actual performance compared to the mean, as a function of the standard deviation. As I described here, we then convert each of these resulting measures, also called z-values, into percentile ranks, using the standard normal distribution.
The second, is that we need to account for the fact that they've each played a different number of seasons. While I used a rather simplistic approach last year, I will present a more sophisticated approach starting with this blog. This method will need further refinement over time, since I am early in my thought process regarding this particular topic. However, it is an improvement over last year's simplistic approach. Actually, the method used last year (average deviation) really doesn't take into account the number of years at all. When I evaluated Brett Favre's career, I simply compared him to a group of quarterbacks who had played a like number of years, and simply ignored any comparisons to players who played far fewer seasons. That method won't work in this case, as we need to compare three quarterbacks with three vastly different career lengths. All else being equal, and they rarely, if ever, are, a player who has a 10 year career versus a player who has a 7 year career should get a higher rating (even if, and especially if, they both had the exact same ratings in every year they played). But the question becomes, "how much higher"?
The third, is that we need to somehow account for the fact that each of the three quarterbacks has played not only a different number of seasons, but that those seasons represent different parts of their careers. In some respects, since the last 8 seasons have been common to all three, we could simply look at the last 8 seasons. But, that would mean that we would be comparing Brett Favre in his 11th through 18th seasons, Peyton Manning through his 5th through 12th seasons, and Drew Brees his first 8 seasons. As I have shown in the past, the early years for a quarterback tend to be the "learning years", and hence this comparison would be inappropriate without the proper adjustments. Brett Favre is also 40 years old, and we have seen declining performances as quarterbacks age (actually, their performances increase at first as they gain experience, and then subsequently decline as the physical toll takes effect). One method is to look at each player's first 8 years, since in that case the comparisons would be over the identical portions of their careers. And perhaps we could compare Favre and Manning for the first 12 years.
Last, we need not assume that the NFL Passer Rating is the measure to be used to evaluate who might be the better passer. The NFL Passer Rating system has a lot of flaws. I will use two measurement methods. The first is the concept of Adjusted Passer Rating. As I have previously mentioned, the average NFL Passer Rating has been steadily increasing every year. The Adjusted Passer Rating normalizes the passer rating so that the average for each year is 66.7. This is then very helpful in evaluating players who played in different years (such as the case here). Last year, I introduced a simple measure of passing efficiency - CMI. I now call this CMTI (or, simplistically, Completions Minus Thrice Interceptions). It is not only simple, but it has a 86% correlation with the NFL Passer Rating. And, because it is so powerful in explaining any variances in the NFL Passer Rating, and because it is so easy to compute, use and explain, I will use it here.
So, to recap, we will make the following adjustments:
- Relate performance in given year to the mean, and then in relation to standard deviation for that year - then convert to percentile rank
- Use a 'better' measure than NFL Passer Rating. We will show that Adjusted NFL Passer Rating is 'better', and CMTI is simpler
- Take into account differing number of seasons played by each quarterback
- Take into account quarterbacks who are at different stages of their career
Here are the respective #s for each quarterback by season:
Brett Favre
Drew Brees
Peyton Manning
What do these performance measures say? Well, there's a lot of information here, so let's take these one at a time.
The first is that the career averages shown (actually all of the averages shown) are simple arithmetic averages of each of the years, not a true weighted average. You can see that these simple averages are close enough to the true average by looking at the career average NFL passer ratings shown in these three exhibits and compare them to the career average for each of the quarterbacks shown earlier.
The second is that regardless of what measure we use, whether it is the NFL Passer Rating, Adjusted NFL Passer Rating, CMTI, first 8 years, last 12 years or what not, it should be very clear that Peyton Manning has performed at a truly superior level compared to the other 2 players (or anyone else for that matter). Just look at CMTI over the past 8 years, for example. He has averaged being in the 90th percentile over the past 8 years. Basically, that suggests that he is, on average, in the Top 3 every year. Truly remarkable. In Peyton Manning, we are watching one of the all-time best. A once-in-a-generation quarterback. In case you didn't notice, the rows highlighted in green indicate a performance that is in the 85th percentile or higher. The areas in blue are 95th percentile or higher.
Ok, Manning is best. That was easy. Now comes the difficult part. Who's better, Favre or Brees?
The case for Favre:
On an Adjusted Passer Rating basis, Brett Favre's first 8 years are comparable to, if not better than Drew Brees' first 8 years (70th percentile versus 68th percentile). We can't directly compare their two careers since one has had an eighteen year career while the other only eight. It is very evident that, but for the last year, Favre's performance has been quite pedestrian over the past 10 years. Well, we can't necessarily penalize him for that, because we don't know how Brees might do in his next 10 years. We don't even know if he is likely to play another 10 years. Given history, however, it is fairly safe to assume that he won't play another 10 years. In my database, going back to 1932, and including both the AAFC in the 1940's as well as the AFL in the 1960's, I have a total of 416 quarterbacks who qualified in at least 1 year. 206 of these quarterbacks qualified in only 1 or 2 years. The average is 4.125 years. There are only 6 quarterbacks that qualified in 15 or more years. Y.A. Tittle qualified in fifteen different seasons. John Elway, Dan Marino and Johnny Unitas each qualified in sixteen different years. And then there are the ultimate marathon men, Fran Tarkenton and Brett Favre, who both qualified on 18 different occasions.
Michael J. Schell, in his book Baseball's All-Time Best Hitters, makes an adjustment to player batting averages, called a late-career adjustment. In essence, he recognizes that a player's performance declines as he ages, and so he makes an adjustment for it. Schell basically caps the at-bats of a player at 8,000. All at-bats beyond 8,000 are ignored. This way, he can compare each player through their first 8,000 at-bats. Doing that type of adjustment would be extremely difficult in the case of football. We know that the average number of passes attempted by a player per game has increased over time (see here and here), so limiting to a fixed number of attempts would be difficult and meaningless at best.
We could limit the player performances to a given number of seasons. Look at the three graphs below.
The first is the average percentile rank of CMTI for all 416 quarterbacks by age.
And the second is the same as the first, except with the x-axis denoting the number of seasons in which they qualified instead of their age.
It appears that there is a learning curve at first (and an elimination process, as presumably the worst players end up having shorter careers), followed by a decline after about 12 years. Keep in mind that this first graph shows the career percentile rank. What we're really interested in is does performance decline as a player ages? So, what we really want to see is the average percentile rank for a given year of a player's career no matter how long they have played.
This is what the third graph shows. It's actually a bit surprising. There doesn't appear to be a significant drop-off. As a matter of fact, out in the 15th-18th years, their performance seems to have got better! Ok, so in the outer years, we're not talking about a lot of players (for years 17 and 18 its just 2 players - Tarkenton and Favre), so anomalies should be expected. So what do we do?
I mentioned earlier that the average number of years in which a quarterback qualifies is 4.125 for all 416 quarterbacks. The table below shows the data in more detail.
There are a total of 77 quarterbacks who have played (qualified) in 8 or more years. That would be 77/416 = 18.5% of the eligible list. The average number of years for this group is 10.8, and the median number of years for this group is 11. It would seem reasonable then to look at a minimum of 8 years as a cut-off. But how do we recognize players who have played a lot longer? The method that I have chosen is to look at a given player's best 8 years. This means several things. First, the obvious - it eliminates from consideration any player who has qualified in fewer than 8 years. Second, it accounts for the fact that players have played longer, and, that fact doesn't hurt them. As a matter of fact, it's the opposite. The less a player has played, the more the "penalty". Third, given the small sample of players playing beyond 14 years, it is quite possible that one or more of them had good years in those later years, so why exclude those years?
So, going back to the tables where I showed each of the three quarterbacks and their individual seasons, you can see that Favre's best 8 years are better than Brees' 8 years. This gives credit to Favre's lengthy career. Remember, less than 20% of the qualified quarterbacks even get to 8 years total, let alone be fortunate enough to have 8 good years. Perhaps over time, Brees might very well exceed Favre. And, if and when he does, we'll give him credit for it at that time.
The case for Brees:
Although comparable to Favre in his first 8 years based on Adjusted Passer Rating, using CMTI gives Brees the nod. Also notice that Brees has had four years where he was better than 85th percentile, while Favre only had one. Brees' last four years in New Orleans have been especially strong. In each of years 5-8, Brees outperforms Favre, while in the first four, Favre outdoes Brees 3 out of four years. Favre, it appears, built his legend largely on the success of his first year, and perhaps even starting with his first game. Check out the following chart.
It shows the average percentile rank for all quarterbacks who played longer than 8 years, for each year they played. Actually, it's not quite the number of years they played in as much as it is the number of years in which they threw enough passes to qualify to have a rating. It's the blue line, and it includes data from the previously mentioned group of 77 quarterbacks. The blue line in this graph is different than the line shown on the third graph earlier, in that this only includes data for those quarterbacks qualifying in 8 or more years. The red line is the data for Drew Brees. The green line is the data for Favre. Clearly, outside of his first year, and then his 3 MVP years (years 3-5 for him), Favre hugged the average for this group of quarterbacks. Some years he did better, and some he did worse.
We're almost done. One more graph.
This one puts a slight twist on the notion of the best years. Instead of just looking at the best 8 years, why don't we look at the best n years, for each year n that the quarterback has played. So this way, we can look at the best year for each quarterback, the best two years, the best three years, and so on. For good measure, I also threw in Manning's #s.
So what do we conclude from all of this?
Again, if there, was any doubt before, this should put it to rest. Manning is clearly the best.
Between Favre and Brees, an argument could be made for either. In terms of a total career, Favre gets the nod, simply because of the number of years he's played. Brees has put up some impressive numbers so far. If he continues this for a few more years, he will most likely finish ahead of Favre, but that remains to be seen. Based on the evidence so far however, he is doing at least as well as, if not better than Favre did in his first 8 years. Perhaps we'll check-in in a few years when Brees retires. And, speaking of retirement, in my next post, I'll take a look at Kurt Warner's career.